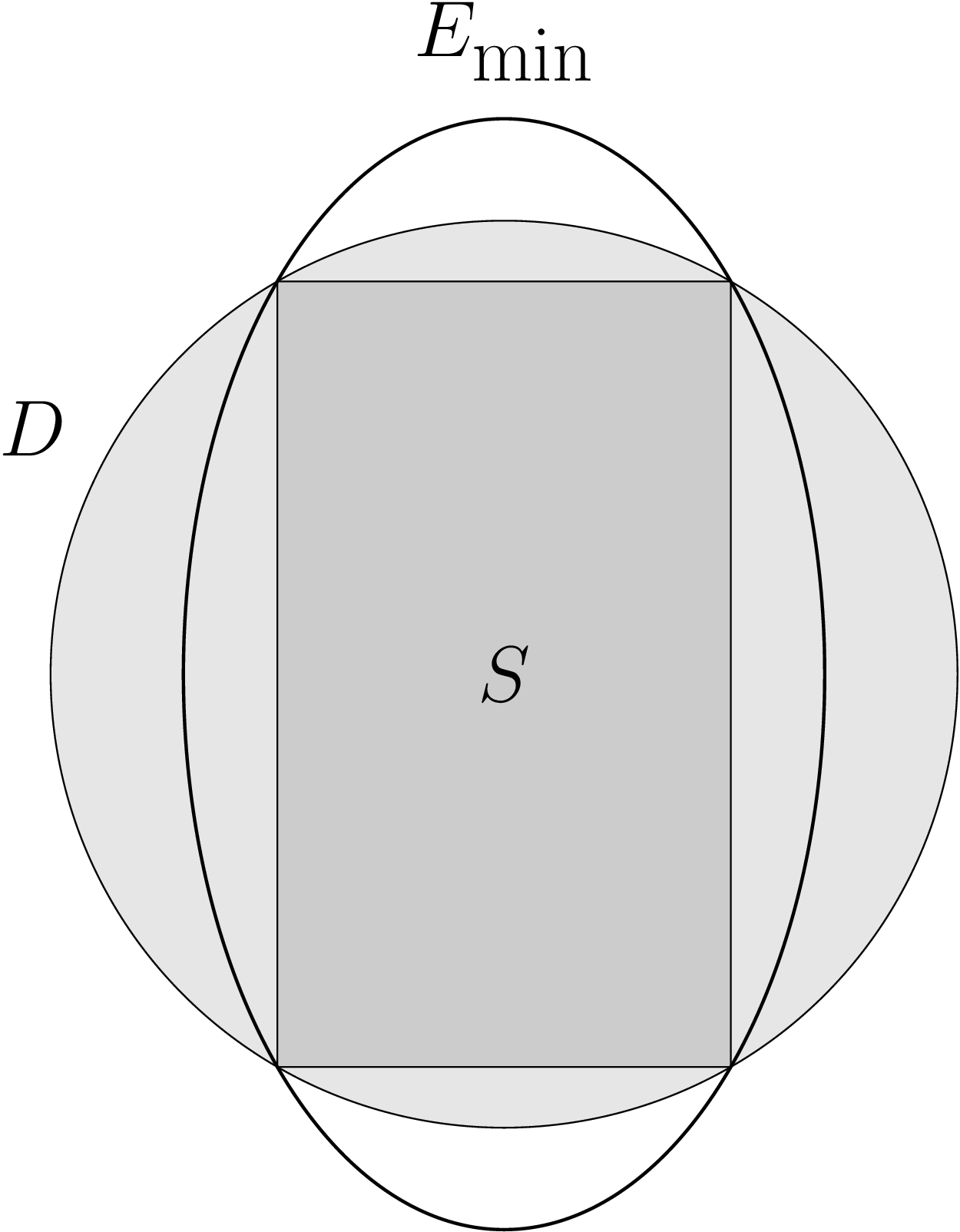My graduate school career (2011-2016) aligned with significant interest within the quantum information community concerning quantifying entanglement. Along with my collaborator, David Dynerman, we explored the set of entangled states from the perspective of orbitopes. This work is unpublished, but more detail can be found in my dissertation. I summarize the results here.

Let $G$ be a group acting on a vector space $V$ and let $v \in V$. Then the convex hull of the orbit of $v$ under the action of $G$ is called an orbitope.

Starting from, for example, the $n$-qubit state $\rho = \vert 0 \cdots 0\rangle \langle 0 \cdots 0\vert $, we can construct the set of separable qubit states as the orbitope generated by the group $SU(2)^n$ under the action $\rho \rightarrow g \rho g^{\dagger}$. Similarly, the of set of all qubit states is generated by the group $SU(2^n)$.

Using a result from Barvinok and Blekherman we can bound the set of separable states within the set of all states using a minimum-volume ellipsoid, effectively creating an approximation of the set of separable states. Furthermore, the polynomial defining this ellipsoid serves as an approximate measure of entanglement, and suggests a structure of entangled states within the set of all states.

This relationship is depicted below, where $S$ is the set of separable states, $D$ is the set of all states, and $E_{\mathrm{min}}$ is the minimum-volume bounding ellipsoid.